
SL Paper 2
The height of water, in metres, in Dungeness harbour is modelled by the function , where is the number of hours after midnight, and and are constants, where and .
The following graph shows the height of the water for hours, starting at midnight.
The first high tide occurs at and the next high tide occurs hours later. Throughout the day, the height of the water fluctuates between and .
All heights are given correct to one decimal place.
Show that .
Find the value of .
Find the value of .
Find the smallest possible value of .
Find the height of the water at .
Determine the number of hours, over a 24-hour period, for which the tide is higher than metres.
OAB is a sector of the circle with centre O and radius , as shown in the following diagram.
The angle AOB is radians, where .
The point C lies on OA and OA is perpendicular to BC.
Show that .
Find the area of triangle OBC in terms of and θ.
Given that the area of triangle OBC is of the area of sector OAB, find θ.
Consider a function , such that , 0 ≤ ≤ 10, .
The function has a local maximum at the point (2, 21.8) , and a local minimum at (8, 10.2).
A second function is given by , 0 ≤ ≤ 10; , .
The function passes through the points (3, 2.5) and (6, 15.1).
Find the period of .
Find the value of .
Hence, find the value of (6).
Find the value of and the value of .
Find the value of for which the functions have the greatest difference.
A water container is made in the shape of a cylinder with internal height cm and internal base radius cm.

The water container has no top. The inner surfaces of the container are to be coated with a water-resistant material.
The volume of the water container is .
The water container is designed so that the area to be coated is minimized.
One can of water-resistant material coats a surface area of .
Write down a formula for , the surface area to be coated.
Express this volume in .
Write down, in terms of and , an equation for the volume of this water container.
Show that .
Find .
Using your answer to part (e), find the value of which minimizes .
Find the value of this minimum area.
Find the least number of cans of water-resistant material that will coat the area in part (g).
The following diagram shows the graph of , for .

The graph of has a minimum point at and a maximum point at .
The graph of is obtained from the graph of by a translation of . The maximum point on the graph of has coordinates .
The graph of changes from concave-up to concave-down when .
(i) Find the value of .
(ii) Show that .
(iii) Find the value of .
(i) Write down the value of .
(ii) Find .
(i) Find .
(ii) Hence or otherwise, find the maximum positive rate of change of .
Let .
Find .
Let . Find .
Olivia’s house consists of four vertical walls and a sloping roof made from two rectangles. The height, , from the ground to the base of the roof is 4.5 m.
The base angles of the roof are and .
The house is 10 m long and 5 m wide.
The length is approximately 2.84 m.
Olivia decides to put solar panels on the roof. The solar panels are fitted to both sides of the roof.
Each panel is 1.6 m long and 0.95 m wide. All the panels must be arranged in uniform rows, with the shorter edge of each panel parallel to or . Each panel must be at least 0.3 m from the edge of the roof and the top of the roof, .
Olivia estimates that the solar panels will cover an area of 29 m2.
Find the length , giving your answer to four significant figures.
Find the total area of the two rectangles that make up the roof.
Find the maximum number of complete panels that can be fitted to the whole roof.
Find the percentage error in her estimate.
Olivia investigates arranging the panels, such that the longer edge of each panel is parallel to or .
State whether this new arrangement will allow Olivia to fit more solar panels to the roof. Justify your answer.
A company is designing a new logo. The logo is created by removing two equal segments from a rectangle, as shown in the following diagram.
The rectangle measures by . The points and lie on a circle, with centre and radius , such that , where . This information is shown in the following diagram.
Find the area of one of the shaded segments in terms of .
Given that the area of the logo is , find the value of .
Using geometry software, Pedro draws a quadrilateral . and . Angle and angle . This information is shown in the diagram.
, where point is the midpoint of .
Calculate the length of .
Show that angle , correct to three significant figures.
Calculate the area of triangle .
Pedro draws a circle, with centre at point , passing through point . Part of the circle is shown in the diagram.
Show that point lies outside this circle. Justify your reasoning.
A Ferris wheel with diameter metres rotates at a constant speed. The lowest point on the wheel is metres above the ground, as shown on the following diagram. is a point on the wheel. The wheel starts moving with at the lowest point and completes one revolution in minutes.
The height, metres, of above the ground after minutes is given by , where .
Find the values of , and .
The following diagram shows a water wheel with centre and radius metres. Water flows into buckets, turning the wheel clockwise at a constant speed.
The height, metres, of the top of a bucket above the ground seconds after it passes through point is modelled by the function
, for .
A bucket moves around to point which is at a height of metres above the ground. It takes seconds for the top of this bucket to go from point to point .
The chord is metres, correct to three significant figures.
Find the height of point above the ground.
Calculate the number of seconds it takes for the water wheel to complete one rotation.
Hence find the number of rotations the water wheel makes in one hour.
Find .
Find .
Determine the rate of change of when the top of the bucket is at .
Two points P and Q have coordinates (3, 2, 5) and (7, 4, 9) respectively.
Let = 6i − j + 3k.
Find .
Find .
Find the angle between PQ and PR.
Find the area of triangle PQR.
Hence or otherwise find the shortest distance from R to the line through P and Q.
The Tower of Pisa is well known worldwide for how it leans.
Giovanni visits the Tower and wants to investigate how much it is leaning. He draws a diagram showing a non-right triangle, ABC.
On Giovanni’s diagram the length of AB is 56 m, the length of BC is 37 m, and angle ACB is 60°. AX is the perpendicular height from A to BC.
Giovanni’s tourist guidebook says that the actual horizontal displacement of the Tower, BX, is 3.9 metres.
Use Giovanni’s diagram to show that angle ABC, the angle at which the Tower is leaning relative to the
horizontal, is 85° to the nearest degree.
Use Giovanni's diagram to calculate the length of AX.
Use Giovanni's diagram to find the length of BX, the horizontal displacement of the Tower.
Find the percentage error on Giovanni’s diagram.
Giovanni adds a point D to his diagram, such that BD = 45 m, and another triangle is formed.
Find the angle of elevation of A from D.
Consider the points A(−3, 4, 2) and B(8, −1, 5).
A line L has vector equation . The point C (5, , 1) lies on line L.
Find .
Find .
Find the value of .
Show that .
Find the angle between and .
Find the area of triangle ABC.
Consider the lines and with respective equations
and .
A third line, , has gradient .
Find the point of intersection of and .
Write down a direction vector for .
passes through the intersection of and .
Write down a vector equation for .
A pan, in which to cook a pizza, is in the shape of a cylinder. The pan has a diameter of 35 cm and a height of 0.5 cm.

A chef had enough pizza dough to exactly fill the pan. The dough was in the shape of a sphere.
The pizza was cooked in a hot oven. Once taken out of the oven, the pizza was placed in a dining room.
The temperature, , of the pizza, in degrees Celsius, °C, can be modelled by
where is a constant and is the time, in minutes, since the pizza was taken out of the oven.
When the pizza was taken out of the oven its temperature was 230 °C.
The pizza can be eaten once its temperature drops to 45 °C.
Calculate the volume of this pan.
Find the radius of the sphere in cm, correct to one decimal place.
Find the value of .
Find the temperature that the pizza will be 5 minutes after it is taken out of the oven.
Calculate, to the nearest second, the time since the pizza was taken out of the oven until it can be eaten.
In the context of this model, state what the value of 19 represents.
The depth of water in a port is modelled by the function , for , where is the number of hours after high tide.
At high tide, the depth is 9.7 metres.
At low tide, which is 7 hours later, the depth is 5.3 metres.
Find the value of .
Find the value of .
Use the model to find the depth of the water 10 hours after high tide.
The diagram shows a circle, centre O, with radius 4 cm. Points A and B lie on the circumference of the circle and AÔB = θ , where 0 ≤ θ ≤ .
Find the area of the shaded region, in terms of θ.
The area of the shaded region is 12 cm2. Find the value of θ.
A restaurant serves desserts in glasses in the shape of a cone and in the shape of a hemisphere. The diameter of a cone shaped glass is 7.2 cm and the height of the cone is 11.8 cm as shown.

The volume of a hemisphere shaped glass is .
The restaurant offers two types of dessert.
The regular dessert is a hemisphere shaped glass completely filled with chocolate mousse. The cost, to the restaurant, of the chocolate mousse for one regular dessert is $1.89.
The special dessert is a cone shaped glass filled with two ingredients. It is first filled with orange paste to half of its height and then with chocolate mousse for the remaining volume.

The cost, to the restaurant, of of orange paste is $7.42.
A chef at the restaurant prepares 50 desserts; regular desserts and special desserts. The cost of the ingredients for the 50 desserts is $111.44.
Show that the volume of a cone shaped glass is , correct to 3 significant figures.
Calculate the radius, , of a hemisphere shaped glass.
Find the cost of of chocolate mousse.
Show that there is of orange paste in each special dessert.
Find the total cost of the ingredients of one special dessert.
Find the value of .
Abdallah owns a plot of land, near the river Nile, in the form of a quadrilateral ABCD.
The lengths of the sides are and angle .
This information is shown on the diagram.

The formula that the ancient Egyptians used to estimate the area of a quadrilateral ABCD is
.
Abdallah uses this formula to estimate the area of his plot of land.
Show that correct to the nearest metre.
Calculate angle .
Find the area of ABCD.
Calculate Abdallah’s estimate for the area.
Find the percentage error in Abdallah’s estimate.
Two straight fences meet at point and a field lies between them.
A horse is tied to a post, , by a rope of length metres. Point is on one fence and point is on the other, such that and radians. This is shown in the following diagram.
The length of the arc shown in the diagram is .
A new fence is to be constructed between points and which will enclose the field, as shown in the following diagram.
Point is due west of and . The bearing of from is .
Write down an expression for in terms of .
Show that the area of the field that the horse can reach is .
The area of field that the horse can reach is . Find the value of .
Hence, find the size of .
Find the size of .
Find the length of new fence required.
Note: In this question, distance is in millimetres.
Let , for .
The graph of passes through the origin. Let be any point on the graph of with -coordinate , where . A straight line passes through all the points .
Diagram 1 shows a saw. The length of the toothed edge is the distance AB.

The toothed edge of the saw can be modelled using the graph of and the line . Diagram 2 represents this model.

The shaded part on the graph is called a tooth. A tooth is represented by the region enclosed by the graph of and the line , between and .
Show that .
Find the coordinates of and of .
Find the equation of .
Show that the distance between the -coordinates of and is .
A saw has a toothed edge which is 300 mm long. Find the number of complete teeth on this saw.
A farmer is placing posts at points , , and in the ground to mark the boundaries of a triangular piece of land on his property.
From point , he walks due west metres to point .
From point , he walks metres on a bearing of to reach point .
This is shown in the following diagram.
The farmer wants to divide the piece of land into two sections. He will put a post at point , which is between and . He wants the boundary to divide the piece of land such that the sections have equal area. This is shown in the following diagram.
Find the distance from point to point .
Find the area of this piece of land.
Find .
Find the distance from point to point .
Let , be a periodic function with
The following diagram shows the graph of .
There is a maximum point at A. The minimum value of is −13 .
A ball on a spring is attached to a fixed point O. The ball is then pulled down and released, so that it moves back and forth vertically.
The distance, d centimetres, of the centre of the ball from O at time t seconds, is given by
Find the coordinates of A.
For the graph of , write down the amplitude.
For the graph of , write down the period.
Hence, write in the form .
Find the maximum speed of the ball.
Find the first time when the ball’s speed is changing at a rate of 2 cm s−2.
The six blades of a windmill rotate around a centre point . Points and and the base of the windmill are on level ground, as shown in the following diagram.
From point the angle of elevation of point is radians.
An observer walks metres from point to point .
The observer keeps walking until he is standing directly under point . The observer has a height of metres, and as the blades of the windmill rotate, the end of each blade passes metres over his head.
One of the blades is painted a different colour than the others. The end of this blade is labelled point . The height , in metres, of point above the ground can be modelled by the function , where is in seconds and . When , point is at its maximum height.
Given that point is metres from the base of the windmill, find the height of point above the ground.
Find the angle of elevation of point from point .
Find the length of each blade of the windmill.
Find the value of and the value of .
If the observer stands directly under point for one minute, point will pass over his head times.
Find the value of .
The following diagram shows quadrilateral ABCD.
Find DB.
Find DC.
Let for .
Let .
The function can be written in the form .
The range of is ≤ ≤ . Find and .
Find the range of .
Find the value of and of .
Find the period of .
The equation has two solutions where ≤ ≤ . Find both solutions.
Adam sets out for a hike from his camp at point A. He hikes at an average speed of 4.2 km/h for 45 minutes, on a bearing of 035° from the camp, until he stops for a break at point B.
Adam leaves point B on a bearing of 114° and continues to hike for a distance of 4.6 km until he reaches point C.
Adam’s friend Jacob wants to hike directly from the camp to meet Adam at point C .
Find the distance from point A to point B.
Show that is 101°.
Find the distance from the camp to point C.
Find .
Find the bearing that Jacob must take to point C.
Jacob hikes at an average speed of 3.9 km/h.
Find, to the nearest minute, the time it takes for Jacob to reach point C.
The quadrilateral ABCD represents a park, where , and . Angle DAB is 70° and angle DCB is 110°. This information is shown in the following diagram.

A straight path through the park joins the points B and D.
A new path, CE, is to be built such that E is the point on BD closest to C.
The section of the park represented by triangle DCE will be used for a charity race. A track will be marked along the sides of this section.
Find the length of the path BD.
Show that angle DBC is 48.7°, correct to three significant figures.
Find the area of the park.
Find the length of the path CE.
Calculate the total length of the track.
An archaeological site is to be made accessible for viewing by the public. To do this, archaeologists built two straight paths from point A to point B and from point B to point C as shown in the following diagram. The length of path AB is 185 m, the length of path BC is 250 m, and angle is 125°.
The archaeologists plan to build two more straight paths, AD and DC. For the paths to go around the site, angle is to be made equal to 85° and angle is to be made equal to 70° as shown in the following diagram.
Find the distance from A to C.
Find the size of angle .
Find the size of angle .
Find the size of angle .
The length of path AD is 287 m.
Find the area of the region ABCD.
Farmer Brown has built a new barn, on horizontal ground, on his farm. The barn has a cuboid base and a triangular prism roof, as shown in the diagram.
The cuboid has a width of 10 m, a length of 16 m and a height of 5 m.
The roof has two sloping faces and two vertical and identical sides, ADE and GLF.
The face DEFL slopes at an angle of 15° to the horizontal and ED = 7 m .
The roof was built using metal supports. Each support is made from five lengths of metal AE, ED, AD, EM and MN, and the design is shown in the following diagram.
ED = 7 m , AD = 10 m and angle ADE = 15° .
M is the midpoint of AD.
N is the point on ED such that MN is at right angles to ED.
Farmer Brown believes that N is the midpoint of ED.
Calculate the area of triangle EAD.
Calculate the total volume of the barn.
Calculate the length of MN.
Calculate the length of AE.
Show that Farmer Brown is incorrect.
Calculate the total length of metal required for one support.
The following diagram shows a semicircle with centre and radius . Points and lie on the circumference of the circle, such that and , where .
Given that the areas of the two shaded regions are equal, show that .
Hence determine the value of .
A farmer owns a plot of land in the shape of a quadrilateral ABCD.
and angle .

The farmer wants to divide the land into two equal areas. He builds a fence in a straight line from point B to point P on AD, so that the area of PAB is equal to the area of PBCD.
Calculate
the length of BD;
the size of angle DAB;
the area of triangle ABD;
the area of quadrilateral ABCD;
the length of AP;
the length of the fence, BP.
The base of an electric iron can be modelled as a pentagon ABCDE, where:

Insulation tape is wrapped around the perimeter of the base of the iron, ABCDE.
F is the point on AB such that . A heating element in the iron runs in a straight line, from C to F.
Write down an equation for the area of ABCDE using the above information.
Show that the equation in part (a)(i) simplifies to .
Find the length of CD.
Show that angle , correct to one decimal place.
Find the length of the perimeter of ABCDE.
Calculate the length of CF.
The following diagram shows a circle with centre and radius .
Points , and lie on the circumference of the circle.
Chord has length and radians.
Show that arc has length .
Show that .
Arc is twice the length of chord .
Find the value of .
All lengths in this question are in centimetres.
A solid metal ornament is in the shape of a right pyramid, with vertex and square base . The centre of the base is . Point has coordinates and point has coordinates .
The volume of the pyramid is , correct to three significant figures.
Find .
Given that , find .
Find the height of the pyramid, .
A second ornament is in the shape of a cuboid with a rectangular base of length , width and height . The cuboid has the same volume as the pyramid.
The cuboid has a minimum surface area of . Find the value of .
A scientist conducted a nine-week experiment on two plants, and , of the same species. He wanted to determine the effect of using a new plant fertilizer. Plant was given fertilizer regularly, while Plant was not.
The scientist found that the height of Plant , at time weeks can be modelled by the function , where .
The scientist found that the height of Plant , at time weeks can be modelled by the function , where .
Use the scientist’s models to find the initial height of
Plant .
Plant correct to three significant figures.
Find the values of when .
For , find the total amount of time when the rate of growth of Plant was greater than the rate of growth of Plant .
At Grande Anse Beach the height of the water in metres is modelled by the function , where is the number of hours after 21:00 hours on 10 December 2017. The following diagram shows the graph of , for .

The point represents the first low tide and represents the next high tide.
How much time is there between the first low tide and the next high tide?
Find the difference in height between low tide and high tide.
Find the value of ;
Find the value of ;
Find the value of .
There are two high tides on 12 December 2017. At what time does the second high tide occur?
A factory packages coconut water in cone-shaped containers with a base radius of 5.2 cm and a height of 13 cm.
The factory designers are currently investigating whether a cone-shaped container can be replaced with a cylinder-shaped container with the same radius and the same total surface area.
Find the volume of one cone-shaped container.
Find the slant height of the cone-shaped container.
Show that the total surface area of the cone-shaped container is 314 cm2, correct to three significant figures.
Find the height, , of this cylinder-shaped container.
The factory director wants to increase the volume of coconut water sold per container.
State whether or not they should replace the cone-shaped containers with cylinder‑shaped containers. Justify your conclusion.
A communication tower, T, produces a signal that can reach cellular phones within a radius of 32 km. A straight road passes through the area covered by the tower’s signal.
The following diagram shows a line representing the road and a circle representing the area covered by the tower’s signal. Point R is on the circumference of the circle and points S and R are on the road. Point S is 38 km from the tower and RŜT = 43˚.
Let SR = . Use the cosine rule to show that .
Hence or otherwise, find the total distance along the road where the signal from the tower can reach cellular phones.
The following diagram shows a circle with centre and radius metres.
Points and lie on the circle and radians.
Find the length of the chord .
Find the area of the shaded sector.
The diagram below shows a triangular-based pyramid with base .
Edge is perpendicular to the edges and .
, , , ,
Calculate
The following diagram shows a triangle ABC.

50° and 112°
Find BC.
Find the area of triangle ABC.
A flat horizontal area, ABC, is such that AB = 100 m , BC = 50 m and angle AĈB = 43.7° as shown in the diagram.
Show that the size of angle BÂC is 20.2°, correct to 3 significant figures.
Calculate the area of triangle ABC.
Find the length of AC.
A vertical pole, TB, is constructed at point B and has height 25 m.
Calculate the angle of elevation of T from, M, the midpoint of the side AC.
The following diagram shows a circle with centre O and radius 40 cm.

The points A, B and C are on the circumference of the circle and .
Find the length of arc ABC.
Find the perimeter of sector OABC.
Find the area of sector OABC.
Let . The line is tangent to the graph of at .
can be expressed in the form r u.
The direction vector of is .
Find the gradient of .
Find u.
Find the acute angle between and .
Find .
Hence, write down .
Hence or otherwise, find the obtuse angle formed by the tangent line to at and the tangent line to at .
The following diagram shows the chord [AB] in a circle of radius 8 cm, where .

Find the area of the shaded segment.
The vector equation of line is given by r .
Point P is the point on that is closest to the origin. Find the coordinates of P.
A metal sphere has a radius 12.7 cm.
Find the volume of the sphere expressing your answer in the form , and .
The sphere is to be melted down and remoulded into the shape of a cone with a height of 14.8 cm.
Find the radius of the base of the cone, correct to 2 significant figures.
A rocket is travelling in a straight line, with an initial velocity of m s−1. It accelerates to a new velocity of m s−1 in two stages.
During the first stage its acceleration, m s−2, after seconds is given by , where .
The first stage continues for seconds until the velocity of the rocket reaches m s−1.
Find an expression for the velocity, m s−1, of the rocket during the first stage.
Find the distance that the rocket travels during the first stage.
During the second stage, the rocket accelerates at a constant rate. The distance which the rocket travels during the second stage is the same as the distance it travels during the first stage.
Find the total time taken for the two stages.
The following diagram shows a right-angled triangle, , with , and .
The points and lie on .
is perpendicular to .
is the arc of a circle, centred at .
The region is bounded by , and arc .
Find .
Find the area of .
The following diagram shows a circle, centre O and radius mm. The circle is divided into five equal sectors.
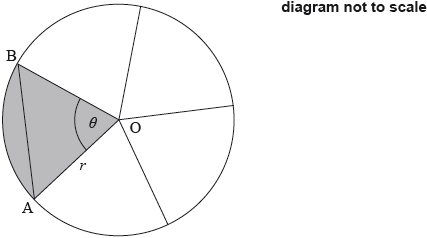
One sector is OAB, and .
The area of sector AOB is .
Write down the exact value of in radians.
Find the value of .
Find AB.
The following diagram shows a circle with centre and radius . Points and lie on the circumference of the circle and , where .
The tangents to the circle at and intersect at point .
Show that .
Find the value of when the area of the shaded region is equal to the area of sector .
The following diagram shows the quadrilateral ABCD.
AB = 6.73 cm, BC = 4.83 cm, BĈD = 78.2° and CD = 3.80 cm.
Find BD.
The area of triangle ABD is 18.5 cm2. Find the possible values of θ.
The following diagram shows part of a circle with centre O and radius 4 cm.
Chord AB has a length of 5 cm and AÔB = θ.
Find the value of θ, giving your answer in radians.
Find the area of the shaded region.
Consider a triangle , where and .
Find the smallest possible perimeter of triangle .
Triangle ABC has a = 8.1 cm, b = 12.3 cm and area 15 cm2. Find the largest possible perimeter of triangle ABC.